On the anatomy of round shapes

Our eyes have been developed to see shapes with their lights and shadows, because we receive information about space in that way. Most of us appreciate Platonic basic solids as the most beautiful shapes, because beauty independent of us is shown in them. (Le Corbusier, A View of Architecture 1922).

This kind of structural geometric beauty, which originates at the same source, occurs also in other shapes worked on by man and nature. Indeed, we admire the beauty of snails, pods, funnels and fruits, as well as the practical shape of a bird’s or insect’s nest, or sand dunes changing in an undulating manner, even shapes created by the movement of water…I will present some primary shapes created from curved foldings, which deserve to be noticed and tell a little about the shapes and objects they have been seen to be used in.

The flexible geometry of curved surfaces has not necessarily been discussed in paper design or origami art. Describing basic shapes with angular mass solids can only passably present their essence and structure. But how to describe space and the geometry of structure that penetrates the entire world of shapes and is ideally shapeless.

We may imagine points in space and matter twisting around them without straight interfaces. After all, a football has been sewn into a dodecahedron from flat pieces of leather, but it becomes a regularly round ball filled with air at the same time as its entire tension is directed outward. One of the main duties of structure is to maintain tensions between extremities. Structure is thus not an aesthetic matter or framework related to layout, but rather an element guaranteeing the durability of a shape. If the tension of a hollow shape is directed outward, most of its material is in the outer ring, and if the shape is as if crushed, its tension is directed toward the centre point.

That is what good bakers have tried to achieve: a practical crust structure, in other words, a structure that stays in its shape, and the beautiful shape has then come as a gift. The geometry of solids can be described with straight and curved lines and between the surface’s extremities. If a flat envelope or plastic bag is filled with air, it becomes a plump pillow. Completely different shapes are created from the same amount of material and a built blank. A cylinder is a tube open on both sides and much more durable if its seam circles around the shape at the same time as it advances from one end to the other than a comparable shape done with a straight seam.  In the same way, the spirality of grains makes the tree trunk stiffer and more durable. A regular tetra can also easily be folded from a cylinder tube, when its ends are flattened into each at a 90 degree angle. Distribution blanks for tetra sides.

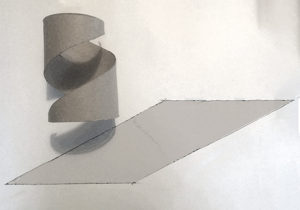
Spirality is connected with nature’s shapes just as with paper shapes. Indeed, the easiest way to create the simplest paper shapes is by twisting strips into funnels and seeing what kinds of blanks and their regularity can be achieved in this manner. Organic shapes of nature grow by increasing their spirality or segmentation in a certain proportion.

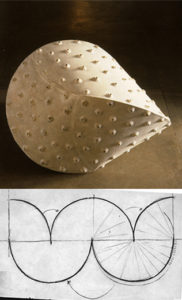
Different simple clam or seed shapes can be created by first using arcs of a circle. With regard to some, it can be seen that the Hellenistic helmet shape applied the shape of a snail, which is obtained by drawing circles that touch one another through the centre point. A shape that has only one continuous surface or side and two edges that are situated in a 90 degree angle toward each other can be obtained from the blank.

On curved basic shapes

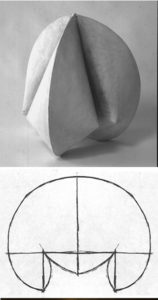
When one draws a circle from paper and cuts open a slash from the edge to the circle’s centre point, the ring of the circle can be folded and the sides twisted on top as a funnel. A few observations can be made of the shape: the higher the funnel, the smaller its ring. If 1/4 of the circle’s ring is folded one on top of another, the funnel’s angle of ascent is 45 degrees. My father made, for example, such a funnel (scoop net) from birch bark, and fastened it to a split willow stem, when he felt thirsty during an outing in the woods.

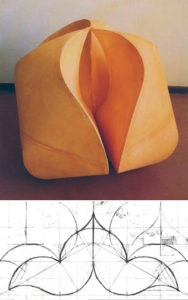
The experiment can be continued also by drawing two circles that intersect each other through their centre points. An oval in the shape of an almond is formed in the middle of the figure. When a blank is cut out along the outline and the oval’s edges are folded outward and the opposite sides of the long outer ring are glued together, the attached segmented shape shaped like a half moon is created. These occur in legumes in different proportions.

 

A third primitive shape is created when a semicircle is drawn and small circles the width of the radius are drawn on both sides of its diameter. Small battens are left in the small circles for the glue. The blank is folded and closed by gluing according to the image.

Furthermore, when the other attached diagram is drawn with the arc of the circle, a snail or clam shape is obtained, which can be closed or left open, whereupon the end result is more organic.

Roundness is relative

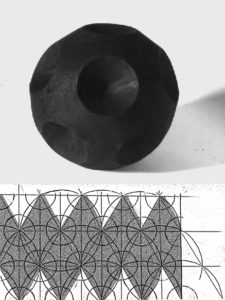
One must remember at all times that we are still dealing with an inelastic surface, which folds in only two directions at the same time, so we will approach roundness with surface geometry. For that reason, the shape of a ball that folds in three directions at the same time is not created by folding paper. Indeed, a student friend once told me that you can certainly making anything from paper, but will you will never be able to make a ball. I thought about this for a while and then realised that if I crumple a thin piece of paper in my hand, I can easily squeeze it into a ball and throw it at that critic. Indeed, the thought of crumpled roundness brings to mind fractals and, on the other hand, 3D graphics. That one can think that a ball’s outline is formed from an extremely crumpled, randomly meandering and folding surface, which looks round in its proportions, because there are so many of its outermost points and they are very close to each other in relation to the ball’s centre point.

Then I began to study the possibility of drawing a blank from one surface. The blank would have funnel-like grooves and its foldings would only consist of the edges of those funnels and the seams could be gathered toward the poles. To achieve the impression of roundness, I had to draw each arc of the circle in a certain proportion and ensure that I can cut inside into the centre point of each circle to be folded. The result was one primary shape with dodecahedron and icosahedron proportions. I understood it only after I had completed the work. Next, I thought already as I was making the sculpture in question, that how about it, if I could build a similar shape from hollow elements, which would consist of a separate inner and outer surface and their sides that are attached to each other. Here are images of a few sculptures, with which I tried that technique. One of the big sculptures that was realised was a work I made from pulp sheets and bible paper for a Finnish-Korean paper art exhibition in Helsinki.

I also studied a free arc element drawn on translucent paper by using the possibility of  folding segmented shapes, from which I realised different pots and paper bottles. In the bottles, I observed how an even surface curved in two directions produces as regular a round shape as possible and what kinds of geometric features it must then have.

I tested discs intersecting each other created from an arc of a circle, from which dishes, buckets or jugs resembling some kind of a pot, in addition to a paper cup blank, could be assembled.

 

Working methods of curved folding

All of the above-mentioned shapes are connected by the fact that they can be realised just as well by free-form arcs as, for example, arcs of a circle.  I use translucent paper to find the necessary arc by drawing, then I find the arc’s smallest repeating element and make it sufficiently symmetric by drawing through and repeating it. When I have the blank’s repeating basic element as a ready tool, I can duplicate it for the use for different blanks. Regular polyhedrons or shapes usually have a geometric polar axis, around which their symmetry has been distributed.

Another working method starts from that anatomical model, which should be reproduced in the same way as how a tailor measures and fits a new piece of clothing according to diagrams. One means is, of course, to use modelling clay or the like, with which one can first make the free shape one desires, and then examine how it could be reproduced and traced as a surface shape according to a diagram that saves material, as long as one remembers that each shape can have many different coats. When making a prototype, one can allow all kinds of errors for oneself, because according to the examined blank, the purpose is to get rid of them in the final work.

In the end, human anatomy can also be seen from a slightly different angle when one thinks of us in shapes of a coat, in which certain angles, tensions, funnels, arcs, deletions, twists etc. are repeated. In no other way than from folded paper can the same kind of sensitivity and beauty be achieved as is in our own anatomical details, or it can if we know how to move what we have learned to another area. For example, one’s own way of drawing can change through paper design, or the shaping of clay can be relearned by first using dry paper, but that is another thing entirely.

A convex shape that curves outward is easy to realise with a few basic foldings, which can be used in anatomical details.  The surface bends into two directions at one time also in these kinds of shapes Attached are a  few completed works, which illustrate this curving of the surface.